Dependence of rate on reactant concentrations

In a first-order reaction, the concentration of reactant decreases from $\text{800}{\text{ mol/dm }}^3{\text{ to 50 mol/dm }}^3$ in$2 \times {10}^2 \mathrm{s}$. The rate constant of reaction in ${\mathrm{s}}^{-1}$ is

Consider a reaction $a\text{G}+\text{bH}\to \text{products}$.  When concentration of both the reactants G and H is doubled, the rate increases by eight times. However, when concentration of G is doubled keeping the concentration of H constant, the rate is doubled. The overall order of the reaction is:

Consider a reaction $a\text{G}+\text{bH}\to \text{products}$.  When concentration of both the reactants $\mathrm{G}$ and $\mathrm{H}$ is doubled, the rate increases by eight times. However, when the concentration of $\mathrm{G}$ is doubled, keeping the concentration of $\mathrm{H}$ constant, the rate is doubled. The overall order of the reaction is:

Consider the chemical reaction:

${\text{N}}_2\left(\text{g}\right)+3{\text{H}}_2\left(\text{g}\right)⟶2{\text{NH}}_3\left(\text{g}\right)$

The rate of this reaction can be expressed in terms of time derivatives of concentration of  $N_2\left(\text{g}\right)\text{ , }{\text{H}}_2\left(\text{g}\right)\text{ and }{\text{NH}}_3\left(\text{g}\right)$ Identify the correct relationship amongst the rate expressions:

What is the principle behind Ostwald's isolation method?

For a zero-order reaction, with the initial reactant concentration $\mathrm{a}$, the time for completion of the reaction is:

Which of the following is incorrect?

The reaction between ${\mathrm{A}}_2$ and ${\mathrm{B}}_2$ follows the equation ${\mathrm{A}}_2+{\mathrm{B}}_2\to 2\mathrm{AB}$. The following data were observed:

The value of the rate constant for the given reaction is:

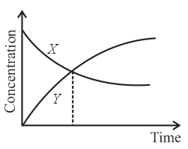
The accompanying figure depicts the change in concentration of species $\mathrm{X}$ and $\mathrm{Y}$ for the elementary reaction $\mathrm{X}\to \mathrm{Y}$ as a function of time. The point of intersection of two curves represents:

The order of a reaction for which a linear line (with a negative slope) is obtained in a graph of $\log (\mathrm{a}-\mathrm{x})$ vs $\mathrm{t}$ is:

Two-third life for a first-order reaction is $55$ minutes. The value of its velocity constant is nearly

The reaction, ${\mathrm{CH}}_3{\mathrm{COOC}}_2{\mathrm{H}}_5\left(\mathrm{aq}\right)+{\mathrm{H}}_2\mathrm{O}\left(\mathrm{l}\right)\stackrel{ }{\to }{\mathrm{CH}}_3\mathrm{COOH}\left(\mathrm{aq}\right)+{\mathrm{C}}_2{\mathrm{H}}_5\mathrm{OH}\left(\mathrm{aq}\right)$ follows the first order kinetics, the rate law being $\mathrm{rate}=\mathrm{k} \left[{\mathrm{CH}}_3{\mathrm{COOC}}_2{\mathrm{H}}_5\right]$. However the reaction shows that, it is second order. Explain.

Consider a reaction that is first order in both directions

$\mathrm{A}\underset{{\mathrm{k}}_{\mathrm{b}}}{\overset{{\mathrm{k}}_{\mathrm{f}}}{\rightleftharpoons }}\mathrm{B}$

Initially only $\mathrm{A}$ is present, and its concentration is ${\mathrm{A}}_0.$ Assume ${\mathrm{A}}_{\mathrm{t}}$ and ${\mathrm{A}}_{\mathrm{eq}}$ are the concentrations of $\mathrm{A}$ at time $"\mathrm{t}"$ and at equilibrium, respectively. The time $"\mathrm{t}"$ at which ${\mathrm{A}}_{\mathrm{t}}=\left({\mathrm{A}}_0+{\mathrm{A}}_{\mathrm{eq}}\right)/2$ is:

For a first order reaction $\mathrm{R}\to \mathrm{P}$, the rate constant is $\mathrm{k}$. If the initial concentration of $\mathrm{R}$ is ${\left[\mathrm{R}\right]}_0$, the concentration of $\mathrm{R}$ at any time $\text{'}\mathrm{t}\text{'}$ is given by the expression:

For a given reaction $3\mathrm{A}\mathrm{ }+\mathrm{B}\to \mathrm{C}+\mathrm{D}$ the rate of reaction can be represented by

For the following elementary reaction, determine its order of reaction and the dimensions of the rate constant:

$2\text{NO}\left(\text{g}\right)\to {\text{N}}_2\text{O}\left(\text{g}\right)+\frac{1}{2}{\text{O}}_2$

The time taken for the completion of three-fourths of a first-order reaction is